RAROC CALCULATIONS
Category: Risk Management in Banking
The RaRoC ratio depends on consistent definitions of the numerator and the denominator.
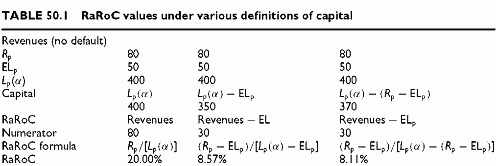
If the revenues are net of expected loss, the capital should also be, otherwise the expected loss would count twice. If the revenues are the contractual revenues, not netting the expected loss, capital should include both expected and unexpected losses. If the revenues net from expected loss serve to absorb losses, capital should be net of expected revenues. Table 50.1 shows examples of alternative calculations of RaRoC. We assume here that the revenues from capital at the risk-free rate, or yf x Kp(a) are included in the total revenue figures.
With revenues netted from capital, the RaRoC ratio is no longer proportional to revenues because revenues are both on the numerator and the denominator. It is easy to increase revenues and observe that all RaRoC ratios still increase with contractual revenues. The RaRoCs based on capital in excess of expected revenues or in excess of ELp are both lower than the RaRoC based on total expected and unexpected loss because the reduction in numerator has more effect than the reduction in denominator. When netting expected
loss from revenues, negative values are possible because the expected loss might be bigger than revenues. In addition, risk-free exposures generate a zero capital, or a negative one when considering revenues! RaRoC is irrelevant in such cases, but it is still possible to determine target revenues in line with profitability goals (see Chapters 53 and 54).
The actual RaRoC values within a mark-to-model framework are more complex than they look. Under full economic valuation, the loss percentile and the ELp both depend on contractual revenues because of the mark-to-model calculation discounting the revenues. Such discounted revenues appear only implicitly in the above ratios because Lp(a)and ELp derive from mark-to-market values that embed revenues. The above equations look oversimplified given that they do not make explicit these underlying interdependencies.
Capital Allocation and Risk Contributions
The standalone risk of a transaction portfolio is intrinsic to a facility and pre-diversification effects within a portfolio. The risk contribution is the risk retained by a facility, or a subportfolio, post-diversification. Risk contributions are the foundation of the capital allocation system and of the risk-adjusted performance measurement system.
Risk contributions are either absolute risk contributions or marginal risk contributions. Marginal risk contributions are the changes in risk with and without an additional unit of exposure, a facility or a subportfolio of facilities. Absolute risk contributions are allocations of the portfolio risk to the existing individual facilities or subportfolios, embedding the correlation structure of the portfolio. Risk contributions are based on such measures as the loss volatility or the portfolio credit risk capital. The loss distribution is a prerequisite for determining risk contributions, since all loss statistics, expected loss, portfolio loss volatility and portfolio capital, derive from the loss distribution.
The mechanisms for calculating risk contributions are similar for market risk and credit risk. They require the loss distributions but do not depend on the actual source of risk. Because of this property, we make no distinction with respect to the nature of risk, market or credit, in this chapter.
Capital allocations are the absolute risk contributions. Only absolute risk contributions add up arithmetically to obtain the overall portfolio risk, while marginal risk contributions do not. Thanks to this attractive property, the risk allocation issue collapses to simple arithmetic operations. Capital allocations are an input to risk-adjusted performance. When considering the existing portfolio only, these measures are ex post, once facilities are within the portfolio. By contrast, marginal risk contributions are relevant for pricing purposes, because they apply well to new transactions. Hence, absolute and marginal risk contributions serve different purposes.
The purpose of this chapter is to define risk contributions, both absolute and marginal risk contributions, and to demonstrate their basic properties. The first section discusses briefly the goals and contributions of the capital allocation system. The second section provides specific definitions of risk contributions. The third section focuses on the key properties of absolute versus marginal risk contributions. Subsequent sections focus on absolute risk contributions and capital allocation only. The fifth section expands a full example of capital allocation, starting from constructing the loss distribution of a simple two-obligor portfolio and ending with the detailed calculations of risk contributions to both loss volatility and risk capital. The next chapter details further marginal risk contributions.